Bulk supplies of single rolls seem the most cost-effective procurement of toilet paper… but what if you could save more money with another buying method?
The Total Cost of Hygiene Consumables
Did you see our other posts in this series? Here’s a quick rundown on the concept.
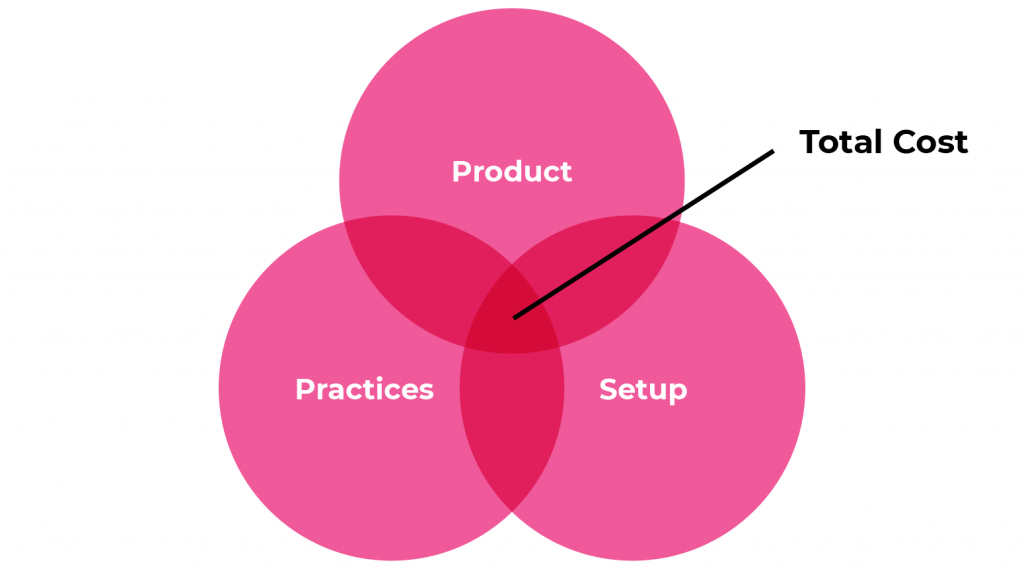
Extensive research in residential aged care has shown us that total cost has three influencing factors:
- Product
- Setup
- Practices
This series aims to show you how total cost works, using simple hygiene consumables case studies.
Total Cost of Toilet Paper
We pulled this case study straight from a site analysis of a large aged care facility on the south coast of New South Wales.
This site had no toilet paper dispensers, instead relying on single roll holders. Staff also placed another roll to the side for when the current roll ran out.

Seems mundane and insignificant, doesn’t it?
Not when you find out that using an alternative product would save the facility $2,783 annually.
Here’s how.
Cost Calculations
First, let’s set up some numbers.
A regular toilet roll has 44m of toilet paper wrapped around its cardboard core.
The alternative coreless rolls have 100m of paper each, and two fit in the recommended dispenser.
One assumption made for this calculation is:
6.84 metres of toilet paper used per day per resident (based on common toilet paper sheet usage numbers), with 10 residents to each toilet location, making 68.4 metres used per day.
| Price (ea) | Length (m) | # | Total Length (m) | Cost by m | Annual cost (ea) | |
| Regular | $1.12 | 44 | 2 | 88 | 1.27c | $316.20 |
| Coreless | $2.25 | 100 | 2 | 200 | 1.25c | $311.22 |
We’ve got a small saving of $4.98 for every replacement of two rolls, annually.
But there’s more.

Time Cost Calculations
For this calculation, we’re assuming:
- average time taken to replace toilet paper = 10 seconds
- required annual changes = 52 (weekly)
- baseline wage = $25.00 per hour.
- 1 change = replacing 2 coreless rolls in a dispenser, and 1 regular roll on a holder
- number of locations requiring toilet paper = 174
(The assumptions above are based on the case study, or on averages from the aged care homes we’ve analysed.)
| Total Length (m) | Weekly Changes (per location) | Locations | Change time | Annual changes | |
| Regular | 88 | 5.44 | 174 | 10s | 49,221.12 |
| Coreless | 200 | 2.39 | 174 | 10s | 21,624.72 |
| Annual time (s) | Annual time (h) | Wage per hour | Annual time cost | Annual total cost | |
| Regular | 492,211 | 136.73 | $25.00 | $3,418.13 | $58,436.93 |
| Coreless | 216,247 | 60.07 | $25.00 | $1,501.72 | $55,654.00 |
Dispensed, coreless toilet paper resulted in over $2,700 in savings for the facility, in an area that few people would regard as costly. See our proofs here.
Factor in more than 60 hours of extra staff time (which could be spent on direct care) and the savings grow exponentially.
The key takeaways from this case study are:
- Total cost is transformed by factors like dispenser capacity (for this example, the length of a toilet roll) and annual refills needed.
- The cost of staff time is the most expensive item on your budget – don’t let it be spent on replacing toilet rolls.
When you’re running a cost analysis, make sure you’ve got all the information you need to make the right decision. Otherwise, you could be unwittingly hurting your facility’s bottom line.
With the exacting standards of hygiene required in aged care, this cost analysis can be applied across all single-use products.
How much time are your staff spending on restocking toilet paper, gloves, masks, or soap cartridges?
Veridia’s Site Survey provides efficiency benchmarking and analysis.
Reach out to us below for more information.
Do you want
to know more?
Reach out one of our Specialists for a time cost analysis.
* Proofs
| Price | Length (m) | # | Total Length (m) | Cost by m | Annual cost (ea) | |
| Regular | a | b | 2 | b x 2 = c | a/c = d | d x 68.4 x 7 x 52 = e |
| Coreless | z | y | 2 | y x 2 = x | z/x = v | v x 68.4 x 7 x 52 = u |
| Total Length (m) | Weekly Changes (per location) | Locations | Change time (s) | Annual changes | |
| Regular | c | (68.4 x 7)/c = f | 174 | 10 | f x 174 x 52 = g |
| Coreless | x | (68.4 x 7)/x = t | 174 | 10 | t x 174 x 52 = s |
| Annual time (s) | Annual time (h) | Wage per hour | Annual time cost | Annual total cost | |
| Regular | g x 10 = h | (h/60)/60 = i | $25.00 | i x 25 = j | j + (e x 174) = k |
| Coreless | s x 10 = r | (r/60)/60 = q | $25.00 | q x 25 = p | p + (u x 174) = o |